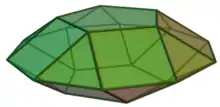
Pentagonal gyrobicupola
In geometry, the pentagonal gyrobicupola is one of the Johnson solids (J31). Like the pentagonal orthobicupola (J30), it can be obtained by joining two pentagonal cupolae (J5) along their bases. The difference is that in this solid, the two halves are rotated 36 degrees with respect to one another.
| Pentagonal gyrobicupola | |
|---|---|
 | |
| Type | Johnson J30 - J31 - J32 |
| Faces | 10 triangles 10 squares 2 pentagons |
| Edges | 40 |
| Vertices | 20 |
| Vertex configuration | 10(3.4.3.4) 10(3.4.5.4) |
| Symmetry group | D5d |
| Dual polyhedron | Elongated pentagonal trapezohedron |
| Properties | convex |
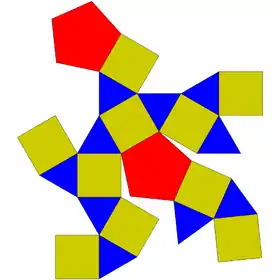
| Net | |
 | |
A Johnson solid is one of 92 strictly convex polyhedra that is composed of regular polygon faces but are not uniform polyhedra (that is, they are not Platonic solids, Archimedean solids, prisms, or antiprisms). They were named by Norman Johnson, who first listed these polyhedra in 1966.[1]
The pentagonal gyrobicupola is the third in an infinite set of gyrobicupolae.
The pentagonal gyrobicupola is what you get when you take a rhombicosidodecahedron, chop out the middle parabidiminished rhombicosidodecahedron (J80), and paste the two opposing cupolae back together.
Formulae
The following formulae for volume and surface area can be used if all faces are regular, with edge length a:[2]
References
- Johnson, Norman W. (1966), "Convex polyhedra with regular faces", Canadian Journal of Mathematics, 18: 169–200, doi:10.4153/cjm-1966-021-8, MR 0185507, Zbl 0132.14603.
- Stephen Wolfram, "Pentagonal gyrobicupola" from Wolfram Alpha. Retrieved July 24, 2010.