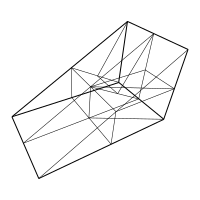
Quaquaversal tiling

The quaquaversal tiling is a nonperiodic tiling of the euclidean 3-space introduced by John Conway and Charles Radin. The basic solid tiles are half prisms arranged in a pattern that relies essentially on their previous construct, the pinwheel tiling. The rotations relating these tiles belong to the group G(6,4) generated by two rotations of order 6 and 4 whose axes are perpendicular to each other. These rotations are dense in SO(3).

References
  • Conway, John H.; Radin, Charles (1998), "Quaquaversal tilings and rotations", Inventiones Mathematicae, 132 (1): 179–188, doi:10.1007/s002220050221, MR 1618635.
  • Radin, Charles; Sadun, Lorenzo (1998), "Subgroups of SO(3) associated with tilings", Journal of Algebra, 202 (2): 611–633, doi:10.1006/jabr.1997.7320, MR 1617675.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.