Steinhaus longimeter

The Steinhaus longimeter, patented by the professor Hugo Steinhaus, is an instrument used to measure the lengths of curves on maps. It is a transparent sheet of three grids, turned against each other by 30 degrees, each consisting of perpendicular lines spaced at equal distances 3.82 mm. The measurement is done by counting crossings of the curve with grid lines. The number of crossings is the approximate length of the curve in millimetres.

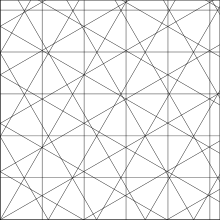
Steinhaus longimeter

The design of the Steinhaus longimeter can be seen as an application of the Crofton formula, according to which the length of a curve equals the expected number of times it is crossed by a random line.[1]

See also
  • Opisometer, a mechanical device for measuring curve length by rolling a small wheel along the curve
  • Pick's theorem, a method for measuring area by counting points

Bibliography
  • Hugo Steinhaus: Mitteilungen der Sächsischen Akad. 82, 120–130, 1930.
  • Hugo Steinhaus: Przeglad Geogr. 21, 1947.
  • Hugo Steinhaus: Comptes Rendus Soc. des Sciences et des Lettres de Wrocław, Sér. B, 1949.
  • Hugo Steinhaus: Mathematical Snapshots, 3rd ed. New York: Dover, pp. 105–110, 1999.

References
  1. Maling, D. H. (2016), Measurements from Maps: Principles and Methods of Cartometry, Butterworth-Heinemann, p. 48, ISBN 9780080984124


This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.