Lens (geometry)
In 2-dimensional geometry, a lens is a convex region bounded by two circular arcs joined to each other at their endpoints. In order for this shape to be convex, both arcs must bow outwards (convex-convex). This shape can be formed as the intersection of two circular disks. It can also be formed as the union of two circular segments (regions between the chord of a circle and the circle itself), joined along a common chord.

Types
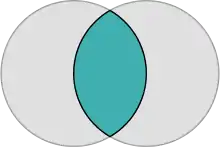
If the two arcs of a lens have equal radius, it is called a symmetric lens, otherwise is an asymmetric lens.
The vesica piscis is one form of a symmetric lens, formed by arcs of two circles whose centers each lie on the opposite arc. The arcs meet at angles of 120° at their endpoints.
Area
- Symmetric
The area of a symmetric lens can be expressed in terms of the radius R and arc lengths θ in radians:
- Asymmetric
The area of an asymmetric lens formed from circles of radii R and r with distance d between their centers is[1]
where
is the area of a triangle with sides d, r, and R.
Applications
A lens with a different shape forms part of the answer to Mrs. Miniver's problem, which asks how to bisect the area of a disk by an arc of another circle with given radius. One of the two areas into which the disk is bisected is a lens.
Lenses are used to define beta skeletons, geometric graphs defined on a set of points by connecting pairs of points by an edge whenever a lens determined by the two points is empty.
See also
- Lune, a related non-convex shape formed by two circular arcs, one bowing outwards and the other inwards
- Lemon, created by a lens rotated around an axis through its tips.[2]
.png.webp)
References
- Weisstein, Eric W. "Lens". MathWorld.
- Weisstein, Eric W. "Lemon". Wolfram MathWorld. Retrieved 2019-11-04.
- Pedoe, D. (1995). "Circles: A Mathematical View, rev. ed". Washington, DC: Math. Assoc. Amer.
- Plummer, H. (1960). An Introductory Treatise of Dynamical Astronomy. York: Dover.
- Watson, G. N. (1966). A Treatise on the Theory of Bessel Functions, 2nd ed. Cambridge, England: Cambridge University Press.
