Gale–Shapley algorithm

In mathematics, economics, and computer science, the Gale–Shapley algorithm (also known as the deferred acceptance algorithm or propose-and-reject algorithm) is an algorithm for finding a solution to the stable matching problem, named for David Gale and Lloyd Shapley who had described it as solving both the college admission problem and the stable marriage problem. It takes polynomial time, and the time is linear in the size of the input to the algorithm. Depending on how it is used, it can find either the solution that is optimal for the participants on one side of the matching, or for the participants on the other side. It is a truthful mechanism from the point of view of the participants for whom it provides the optimal solution.

Background
Main article: stable matching problem

The stable matching problem, in its most basic form, takes as input equal numbers of two types of participants (n men and n women, or n medical students and n internships, for example), and an ordering for each participant giving their preference for whom to be matched to among the participants of the other type. A matching is not stable if:

  1. There is an element A of the first matched set which prefers some given element B of the second matched set over the element to which A is already matched, and
  2. B also prefers A over the element to which B is already matched.

In other words, a matching is stable when there does not exist any match (A, B) which both prefer each other to their current partner under the matching. A stable matching always exists, and the algorithmic problem solved by the Gale–Shapley algorithm is to find one.

Solution
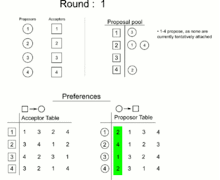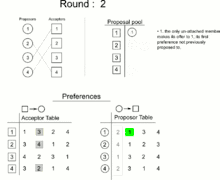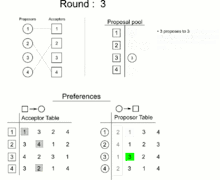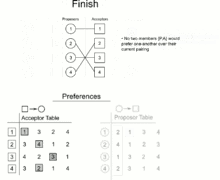
Animation showing an example of the Gale–Shapley algorithm

In 1962, David Gale and Lloyd Shapley proved that, for any equal number of men and women, it is always possible to solve the SMP and make all marriages stable. They presented an algorithm to do so.[1][2] In 1984, Alvin E. Roth observed that essentially the same algorithm had already been in practical use since the early 1950s, in the National Resident Matching Program.[3]

The Gale–Shapley algorithm involves a number of "rounds" (or "iterations"):

  • In the first round, first a) each unengaged man proposes to the woman he prefers most, and then b) each woman replies "maybe" to her suitor she most prefers and "no" to all other suitors. She is then provisionally "engaged" to the suitor she most prefers so far, and that suitor is likewise provisionally engaged to her.
  • In each subsequent round, first a) each unengaged man proposes to the most-preferred woman to whom he has not yet proposed (regardless of whether the woman is already engaged), and then b) each woman replies "maybe" if she is currently not engaged or if she prefers this man over her current provisional partner (in this case, she rejects her current provisional partner who becomes unengaged). The provisional nature of engagements preserves the right of an already-engaged woman to "trade up" (and, in the process, to "jilt" her until-then partner).
  • This process is repeated until everyone is engaged.

The runtime complexity of this algorithm is where is the number of men or women.[4]

This algorithm guarantees that:

Everyone gets married
At the end, there cannot be a man and a woman both unengaged, as he must have proposed to her at some point (since a man will eventually propose to everyone, if necessary) and, being proposed to, she would necessarily be engaged (to someone) thereafter.
The marriages are stable
Let Alice and Bob both be engaged, but not to each other. Upon completion of the algorithm, it is not possible for both Alice and Bob to prefer each other over their current partners. If Bob prefers Alice to his current partner, he must have proposed to Alice before he proposed to his current partner. If Alice accepted his proposal, yet is not married to him at the end, she must have dumped him for someone she likes more, and therefore doesn't like Bob more than her current partner. If Alice rejected his proposal, she was already with someone she liked more than Bob.

Algorithm

 
algorithm stable_matching is
    Initialize all m ∈ M and w ∈ W to free
    while ∃ free man m who still has a woman w to propose to do
        w := first woman on m's list to whom m has not yet proposed
        if w is free then
            (m, w) become engaged
        else some pair (m', w) already exists
            if w prefers m to m' then
                m' becomes free
                (m, w) become engaged 
            else
                (m', w) remain engaged
            end if
        end if
    repeat

Optimality of the solution
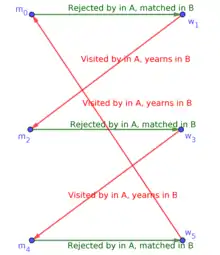
Main article: Lattice of stable matchings
Proof that deferred acceptance is optimal for men

The existence of different stable matchings raises the question: which matching is returned by the Gale-Shapley algorithm? Is it the matching better for men, for women, or the intermediate one? In the above example, the algorithm converges in a single round on the men-optimal solution because each woman receives exactly one proposal, and therefore selects that proposal as her best choice, ensuring that each man has an accepted offer, ending the match.

This is a general fact: the Gale-Shapley algorithm in which men propose to women always yields a stable matching that is the best for all men among all stable matchings. Similarly, if the women propose then the resulting matching is the best for all women among all stable matchings. These two matchings are the top and bottom elements of a larger structure on all stable matchings, the lattice of stable matchings.

To see this, consider the illustration at the right. Let A be the matching generated by the men-proposing algorithm, and B an alternative stable matching that is better for at least one man, say m0. Suppose m0 is matched in B to a woman w1, which he prefers to his match in A. This means that in A, m0 has visited w1, but she rejected him (this is denoted by the green arrow from m0 to w1). w1 rejected him in favor of some man that she prefers, say m2. So in B, w1 is matched to m0 but "yearns" (wants but unmatched) for m2 (this is denoted by the red arrow from w1 to m2).

Since B is a stable matching, m2 must be matched in B to some woman he prefers to w1, say w3. This means that in A, m2 has visited w3 before arriving at w1, which means that w3 has rejected him. By similar considerations, and since the graph is finite, we must eventually have a directed cycle in which each man was rejected in A by the next woman in the cycle, who rejected him in favor of the next man in the cycle. But this is impossible since such "cycle of rejections" cannot start anywhere: suppose by contradiction that it starts at e.g. m0 - the first man rejected by his adjacent woman (w1). By assumption, this rejection happens only after m2 comes to w1. But this can happen only after w3 rejects m2 - contradiction to m0 being the first.

Strategic considerations

The GS algorithm is a truthful mechanism from the point of view of men (the proposing side). I.e, no man can get a better matching for himself by misrepresenting his preferences. Moreover, the GS algorithm is even group-strategy proof for men, i.e., no coalition of men can coordinate a misrepresentation of their preferences such that all men in the coalition are strictly better-off.[5] However, it is possible for some coalition to misrepresent their preferences such that some men are better-off and the other men retain the same partner.[6]

The GS algorithm is non-truthful for the women (the reviewing side): each woman may be able to misrepresent her preferences and get a better match.

Implementation in software packages
  • R: The Gale–Shapley algorithm (also referred to as deferred-acceptance algorithm) for the stable marriage and the hospitals/residents problem is available as part of the matchingMarkets[7][8] and matchingR[9] packages.
  • API: The MatchingTools API provides a free application programming interface for the Gale–Shapley algorithm.[10]
  • Python: The Gale–Shapley algorithm is included along with several other well-known matching game algorithms in the matching library,[11] and QuantEcon/MatchingMarkets.py package[12] includes several others for generalized matching games.
  • MATLAB: The Gale–Shapley algorithm is implemented in the assign2DStable function that is part of the United States Naval Research Laboratory's free Tracker Component Library.[13]

Application

In the 1980s, Roth explored matching between medical interns and hospitals, and showed that the algorithm then in use by the National Resident Matching Program—a clearinghouse that matched residency applicants to hospitals—was successful because it followed principles similar to those encoded by the Gale-Shapley algorithm. In the mid-1990s, Roth was called upon to improve the NRMP algorithm to make matching more efficient and to better accommodate special needs such as placing dual-doctor couples in the same hospital. Roth delivered an improved algorithm, working with Elliott Peranson, the founder of the Toronto, Canada-based company National Matching Services Inc. Shapley and Roth were awarded 2012 Nobel Memorial Prize in Economic Sciences "for the theory of stable allocations and the practice of market design"; Gale had died in 2008.[14]

See also

References
  1. Gale, D.; Shapley, L. S. (1962). "College Admissions and the Stability of Marriage". American Mathematical Monthly. 69 (1): 9–14. doi:10.2307/2312726. JSTOR 2312726.
  2. Harry Mairson: "The Stable Marriage Problem", The Brandeis Review 12, 1992 (online).
  3. Bergstrom, Theodore C. (June 1992). "Review of Two-Sided Matching: A Study in Game-Theoretic Modeling and Analysis by A. E. Roth and M. A. O. Sotomayor". Journal of Economic Literature. 30 (2): 896–898. JSTOR 2727713.
  4. Iwama, Kazuo; Miyazaki, Shuichi (2008). "A Survey of the Stable Marriage Problem and Its Variants" (PDF). International Conference on Informatics Education and Research for Knowledge-Circulating Society (icks 2008): 131–136. doi:10.1109/ICKS.2008.7. hdl:2433/226940. ISBN 978-0-7695-3128-1.
  5. Dubins, L. E.; Freedman, D. A. (1981). "Machiavelli and the Gale–Shapley algorithm". American Mathematical Monthly. 88 (7): 485–494. doi:10.2307/2321753. MR 0628016.
  6. Huang, Chien-Chung (2006). "Cheating by men in the Gale-Shapley stable matching algorithm". In Azar, Yossi; Erlebach, Thomas (eds.). Algorithms - ESA 2006, 14th Annual European Symposium, Zurich, Switzerland, September 11-13, 2006, Proceedings. Lecture Notes in Computer Science. 4168. Springer. pp. 418–431. doi:10.1007/11841036_39. MR 2347162.
  7. Klein, T. (2015). "Analysis of Stable Matchings in R: Package matchingMarkets" (PDF). Vignette to R Package MatchingMarkets.
  8. "matchingMarkets: Analysis of Stable Matchings". R Project.
  9. "matchingR: Matching Algorithms in R and C++". R Project.
  10. "MatchingTools API".
  11. Wilde, H.; Knight, V.; Gillard, J. (2020). "Matching: A Python library for solving matching games". Journal of Open Source Software. doi:10.21105/joss.02169.
  12. "matchingMarkets.py". Python package.
  13. "Tracker Component Library". Matlab Repository. Retrieved January 5, 2019.
  14. "Economics Nobel Honors Perfect Match". Science Mag. Retrieved December 5, 2020.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.