Conoid
In geometry a conoid (Greek: κωνος cone and -ειδης similar) is a ruled surface, whose rulings (lines) fulfill the additional conditions
- (1) All rulings are parallel to a plane, the directrix plane.
- (2) All rulings intersect a fixed line, the axis.
- The conoid is a right conoid, if its axis is perpendicular to its directrix plane. Hence all rulings are perpendicular to the axis.
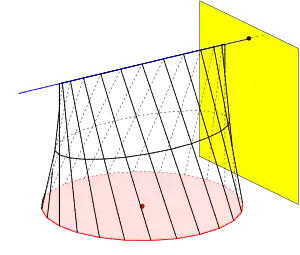
Because of (1) any conoid is a Catalan surface and can be represented parametrically by
Any curve with fixed parameter is a ruling, describes the directrix and the vectors are all parallel to the directrix plane. The planarity of the vectors can be represented by
- .
- If the directrix is a circle the conoid is called circular conoid.
The term conoid was already used by Archimedes in his treatise On conoids and spheroides.
Examples
Right circular conoid
The parametric representation
- describes a right circular conoid with the unit circle of the x-y-plane as directrix and a directrix plane, which is parallel to the y--z-plane. Its axis is the line
Special features:
- The intersection with a horizontal plane is an ellipse.
- is an implicit representation. Hence the right circular conoid is a surface of degree 4.
- Kepler's rule gives for a right circular conoid with radius and height the exact volume: .
The implicit representation is fulfilled by the points of the line , too. For these points there exist no tangent planes. Such points are called singular.
Parabolic conoid
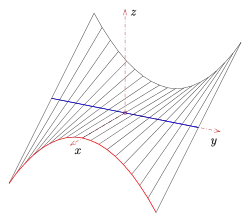
The parametric representation
describes a parabolic conoid with the equation . The conoid has a parabola as directrix, the y-axis as axis and a plane parallel to the x-z-plane as directrix plane. It is used by architects as roof surface (s. below).
The parabolic conoid has no singular points.
Further examples
 hyperbolic paraboloid
hyperbolic paraboloid Plücker conoid
Plücker conoid Whitney umbrella
Whitney umbrella
Applications
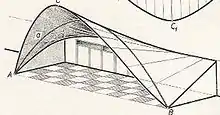
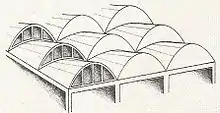
Mathematics
There are a lot of conoids with singular points, which are investigated in algebraic geometry.
Architecture
Like other ruled surfaces conoids are of high interest with architects, because they can be built using beams or bars. Right conoids can be manufactured easily: one threads bars onto an axis such that they can be rotated around this axis, only. Afterwards one deflects the bars by a directrix and generates a conoid (s. parabolic conoid).
External links
- mathworld: Plücker conoid
- "Conoid", Encyclopedia of Mathematics, EMS Press, 2001 [1994]